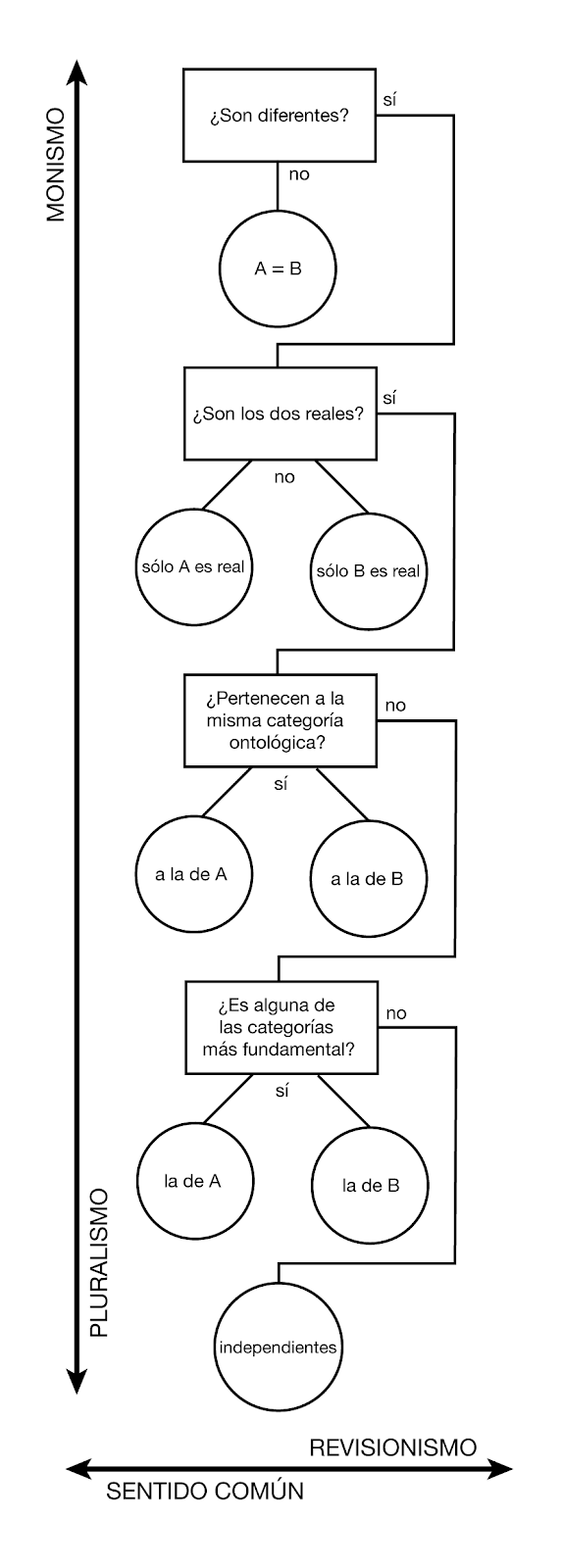
How to visually represent a structure?

 Like any other kind of visual representation, you will need to follow the golden rule: 

use things that are patently related/similar/different
to represent things that are relevantly related/similar/different

1. First, you are going to need to represent the elements in the structure. Use itemised elements like points, geometric figures, letters, etc. This will be your terminal vocabulary, so to speak, corresponding to what Greenberg calls the first order elements of the system and thus can be as iconic or symbolic as desired.
2. Then you are going to need how to represent their properties and relations. The mechanisms you can use to represent them are of two types: intrinsic and extrinsic.
1. Intrinsic [I was very tempted to call them Leibnizian but I think the reference may be too obscure for many. It’s a shame]: You can exploit similarities , relations and differences in the way you have decided to represent the elements in the structure to represent similarities, relations and differences among the elements themselves. For example, using letters of same or similar fonts, color, size, etc. to indicate that the elements represented by those letters are related or of the same kind, while using letters of contrasting  fonts, color, size, etc. to indicate that they are unrelated or of different kinds (Dair 1967). 
   I have called these mechanisms intrinsic, because they pertain to how the element itself is represented and remain there no matter its location or what external elements are added.
2. Extrinsic. These can be of two kinds. 
1. One way is to exploit the spatio-temporal location of the elements. Thus, we can represent two objects as being more similar to a third by locating the (elements that represent the) first two closer to each other than to the third (this is one of the basic principles of composition in film editing, for example; see Dmytkyk 1984). We can also use the center of the page to highlight an element and/or move another to the periphery to represent its lesser status.
   One has to be very careful here, because since space and time re themselves structured it is impossible to represent elements spatiotemporally without automatically representing them spatiotemporally related. If you just write a sequence of two letters, for example, you have already represented them as one being the first and the other the second. Beware!
2. The second way is by introducing new elements to mark the relations or differences between the elements. For example, we can encircle elements that are similar or related, draw a line separating elements that are different, or an arrow joining elements that are related, etc. We can also use parenthesis to group symbols together, and we can also use other typographic marks like underlining or asterisks, as well as shading crossing, etc. We can also (and usually do) use new letters besides the previous letters used to represent the elements to represent their properties and relations. 
   Just like space, in most cases, our marks are also structured themselves and thus using one sort or mark or another might suggest that the relation represent has an analogous structure as the mark. For example, if we enclose a group of elements in a circle, we are not only signaling that those elements are similarly related, but that such relation forms an equivalence class, i.e., it is transitive, symmetric, etc. Similarly, if we link a group of elements pairwise with similar lines – one line linking an element a with an element b and another linking b to c, etc. –, we would again signal that the same relation holds among each pair, but not that such relation is, for example, transitive. And if the line is symmetric (on an axis perpendicular to the elements) we would have also signaled that the relation is symmetric, etc. 
   Also, notice that in formulating the golden rule above I have highlighted the condition "patently", because not any difference, similarity or relation would do. They need to be noticeable and harmonious. This aims to capture a generalisation of Dair's (1967: 66) distinction between contrasting and conflicting differences: For example, if you want to use differences in size to represent differences among your target items, but you make those differences too small, your reader/interpreter might either miss those differences or think they are the result of carelessness. Dair mentions as another example of a conflicting difference that between script and italic letters. I am sure many non-designers cannot even identify the difference between these two typographical categories!
3. On the other hand, of course, if you make them too large, you might waste too much space and create inharmonious designs.

1. Recursion. That is all that is needed actually. Since these mechanisms can be iterated recursively we can get also second order relations and similarities the same way reaching higher in Greenberg's hierarchy. For example, using different sorts of lines – dotted, continuous, wiggly, etc. – to link elements we can represent different sort of relations among them. We can also use lines to link lines that link lines, etc. Using elements of different sizes we can represent hierarchies, etc.
   One of the most useful tools we have devised to allow for the representation of second order relations/similaritites/contrasts etc. is indexing (famously studied by Netz 1998  on Euclidean diagrams) i.e., using more than one mechanism (usually two different sort of marks) to represent the same relation or property in order to multiply the possible similarities that can be exploited. For example, we can use a circle to group some elements and then attach a letter to the circle, so that then we can use both similar letters and/or similar circles to denote that other relations are similar.

If you apply these mechanisms you will get a nice iconic structural representation, since your system will exploit structural similarities between representation and target. As a matter of fact, this is how most (epistemic and artistic) systems of representations operate and that is why they all tend to be iconic at the structural level.

This characterisation has the advantage of showing how diagrams and formulas are actually just two variants of a more general kind. One can also see thus that diagrams like Peirce’s directed graphs and Frege’s conceptual notation stand on a continuum of visual representation of forms along with more so-called formal representations like the usual formulas of contemporary logic of the Polish system of notation. As a matter of fact, we can use this characterisation to give a more rigorous account of what is it for some system to be a mere notational variant of another.